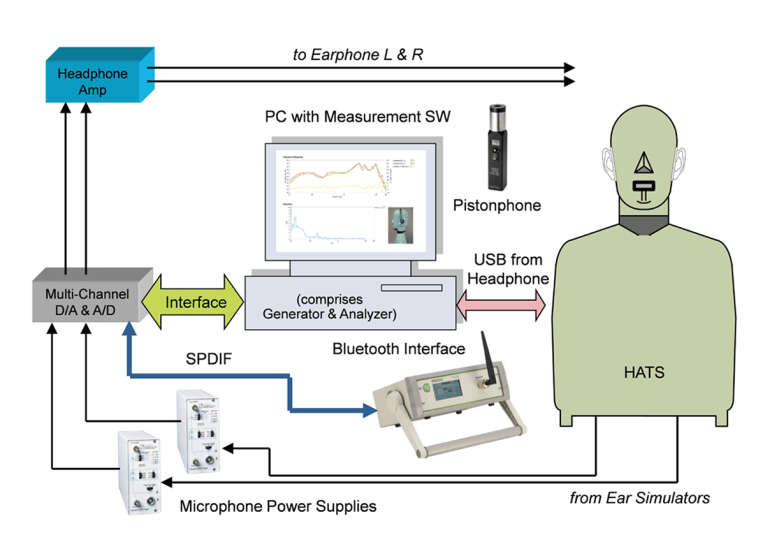
Test System
A complete measurement system for performing comprehensive measurements of headphone systems should consist of the following elements:
• PC with automated test software (includes generator, analyzer and USB interface)
• Soundcard or multichannel D/A and A/D
• Head-and-torso simulator (HATS)
• Headphone amplifier
• Microphone power supplies
• Bluetooth interface
• Pistonphone or calibrator
• Cables
An example of a complete headphone test system is shown in Figure 1.
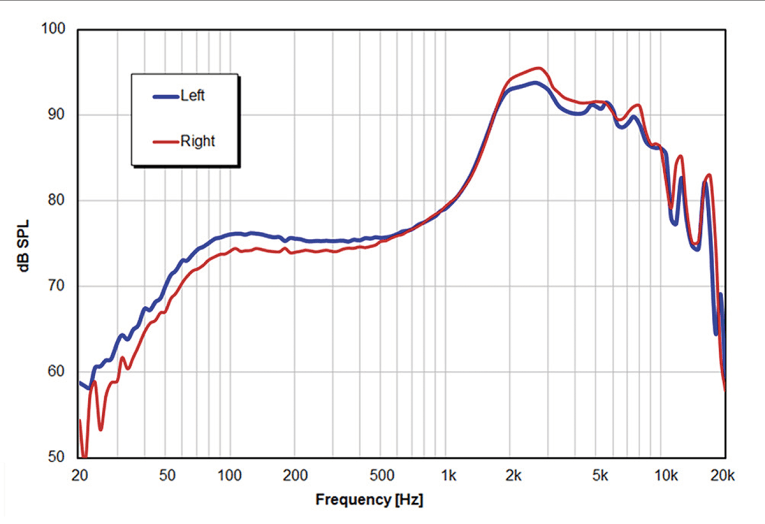
Sensitivity and Frequency Response
Frequency response is measured from 20 Hz to 20 kHz at the ISO R40 (1/12 octave) frequencies using a stepped sine input signal. The frequency response, second and third harmonic distortion can be measured simultaneously. The response is normally plotted as decibels (dB) sound pressure level (SPL) vs. log frequency[1]. An example is shown in Figure 2.
The sensitivity for a passive headphone is calculated as:
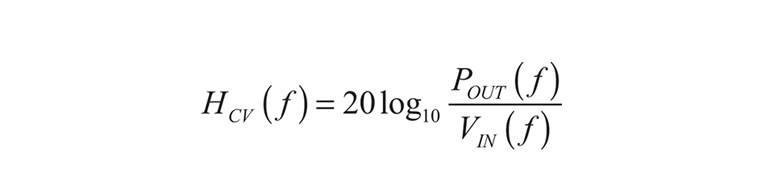
where pOUT is the RMS sound pressure [in Pa] measured in the HATS ear simulator, and VIN is the voltage [in V] at the input terminals of the headphone under test. This can be at single frequency (e.g., 500 Hz) or, at all frequencies in the response. In this case, the decibels of the response have units (e.g., dB Pa/V) and the response at each frequency is the calibrated sensitivity of the device[2]. However, in most cases, the sensitivity simply specified as the output level in dBSPL for a given input level. For Bluetooth and USB devices, the input level is referred to dBFS (e.g., -6 dBFS) and the sensitivity is then dBSPL out for a given test level in dBFS.
The test level for a passive headphone is specified as an input voltage that dissipates 1 mW across a resistive load equal to the nominal impedance. Alternatively, the test may be performed at a given output level (e.g., 94 dBSPL at 500 Hz), however, in this case, the input level should be within the linear range of operation of the device. Either method is repeatable and equally applicable to systems or component transducers. The output level method is typically used for digital or wireless devices.
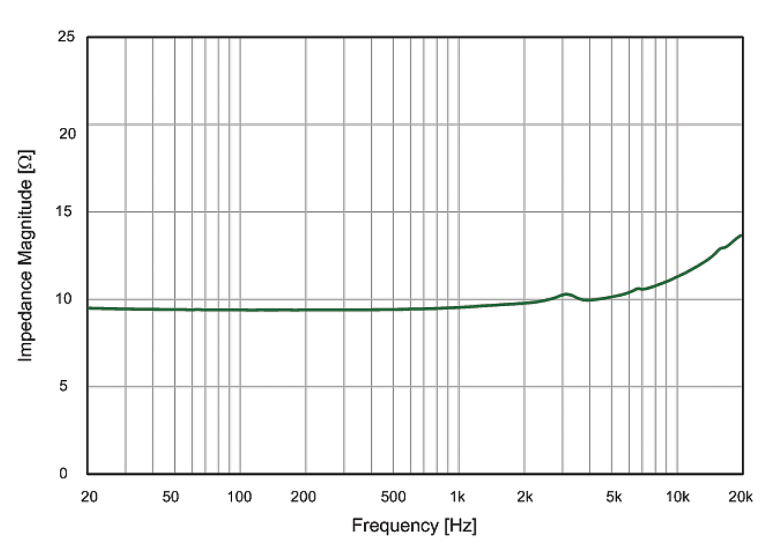
Electrical Impedance
The IEC 60268-7 definition of the nominal impedance is that the minimum value of the electrical impedance modulus (magnitude) vs. frequency, as measured on the HATS, shall be greater than or equal to 80% of the nominal impedance, Z0. The nominal impedance is intended to represent the equivalent resistive load for an input reference power of 1 mW. The specification for the nominal impedance is therefore, effectively:
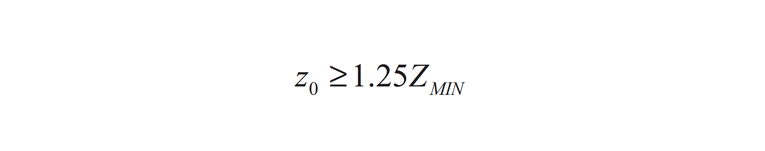
In order to perform headphone frequency response measurements, the input power of 1 mW across Z0 must be converted into an actual generator voltage. A simple algebraic rearrangement of Ohm’s Law yields the required input voltage as:
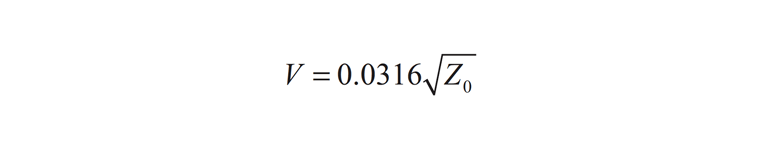
Positioning Errors and Test Repeatability
When a circum-aural, supra-aural, or intra-concha headphone or headset is placed on the HATS and tested, repeatability of the results may be an issue due to variations in positioning, particularly for high acoustic impedance (sealed) devices. Nonetheless, relatively accurate and repeatable results can be obtained by making several measurements and averaging the results[2]. A minimum of 5 frequency response measurements are performed on each device tested (see Figure 4).
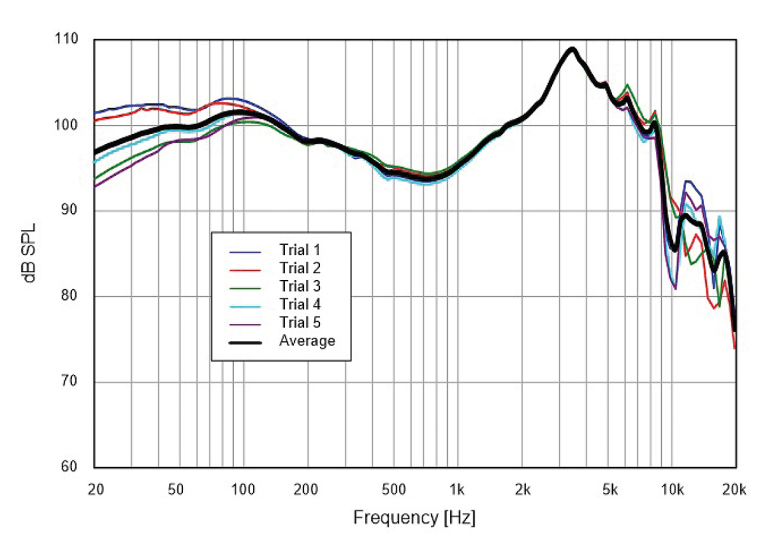
Note the large variations at low frequencies due to leakage and at high frequencies due to slight variations in position. The headphone is completely removed from the HATS and re-mounted for each of the five trials. The mean and standard deviation (in decibels) at each frequency in the response are computed for the five measurement trials.
The reported response is the decibel average of all five trials. The response of the individual trials may also be provided for information purposes. For headphones, the accuracy may be considered to be acceptable if the standard deviation is less than 2 dB from 500 Hz to 5 kHz. Trials outside the standard deviation limits due to miss-positioning may be rejected and the test re-run. Repositioning and averaging is generally not required for insert earphones having only a single repeatable position.
Free and Diffuse Field Corrected Responses
The response of a headphone as measured on a manikin at the ear Drum Reference Point (DRP) does not directly correspond to the perceived listener response. The headphone response must compensate for the head diffraction and ear canal response in the sound field, generally resulting in a non-flat measured response. The measured response can, however, be normalized to a sound field response by subtracting (in decibels) an appropriate target or reference response. The resulting “error function” is the insertion gain of the device. A “flat” insertion gain vs. frequency corresponds to a uniform or flat perceived listener response[3].
The free field as a reference response is equivalent to the response of a median human measured at the ear drum with an ideal point source on-axis, 0° azimuth, and 0° elevation under anechoic free field conditions[3],[4]. This response emphasizes the direct sound from a single direction, and does not consider the reverberant sound field. As a target earphone response, the free field response is unrealistic as it represents anechoic conditions, which are never encountered in a real listening situation.
Alternatively, the diffuse field response has been used as a target earphone response. The rationale is that since the direction of any given playback source is unknown, a random incidence, or diffuse sound field, gives equal emphasis or weighting to sound from all directions[3],[4]. The diffuse field response also represents an unrealistic listening condition, as most real listening rooms are not purely diffuse.
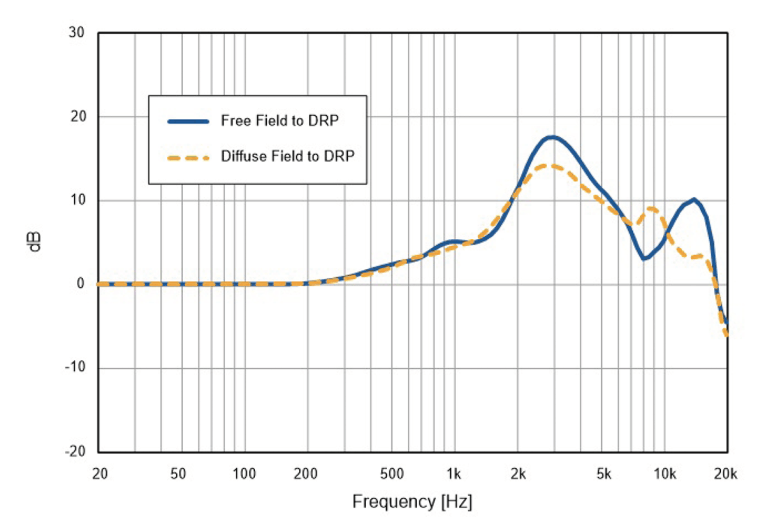
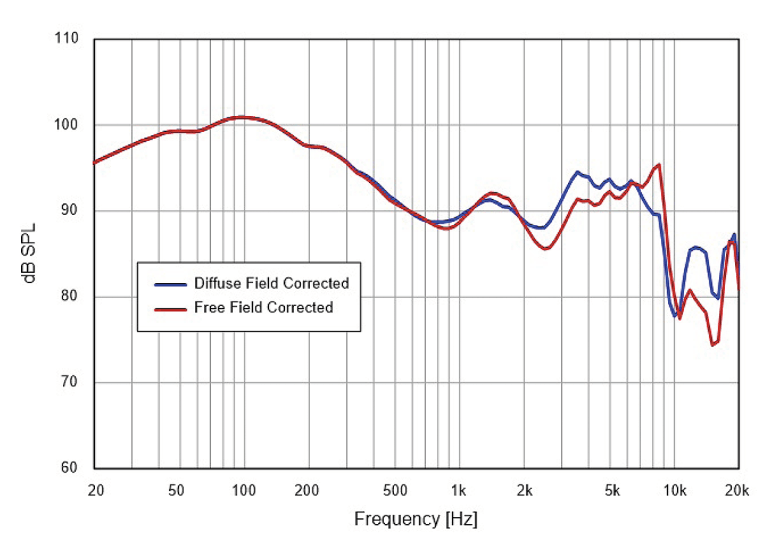
The HATS accurately represents a median adult, its response is standardized, and its free and diffuse response data are readily available. In fact, the standardized test manikin, in addition to being a convenient fixture on which to perform headphone measurements, is actually a physical embodiment of the reference response[5]. Figure 5 shows the IEEE 1652 standard free and diffuse field responses to DRP.
Normalizing or correcting to these standard responses makes the test completely objective – yet provides statistical correlation to the median human response. Figure 6 shows the free and diffuse field corrected responses for the consumer headphone of Figure 4.
This unit does not achieve a flat insertion gain and the 10 dB to 12 dB bass boost centered at 100 Hz is prominent. Not surprisingly, this unit sounds boomy. Calculating both the diffuse and free field corrected responses provides a useful indicator of the perceived sound of a headphone, as well as where its response fits with respect to the median adult human response. Plots of both corrected responses will also indicate if some other alternative target response was used, or none as the case may be.
Left-Right Tracking
For proper stereo balance, it is also important to gauge the sensitivity and the loudness matching of the left and right channels of a stereo headphone. However, simply taking the ratio of the left to right earphone responses at each frequency (or equivalently, the difference in decibels), can result in an uneven plot that is difficult to interpret. Therefore, the Left and Right (L-R) responses can be post-processed to mitigate this problem. Prior to the L-R Tracking calculation, the response in each channel is power averaged in each 1/3 octave band [2]. The power averaged response value in decibels in each 1/3 octave band is calculated as:
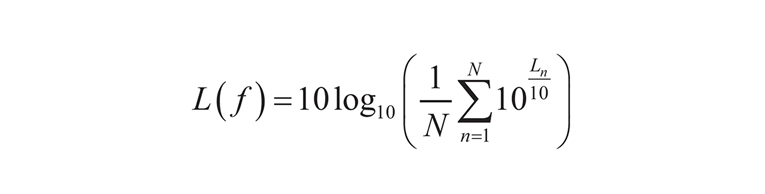
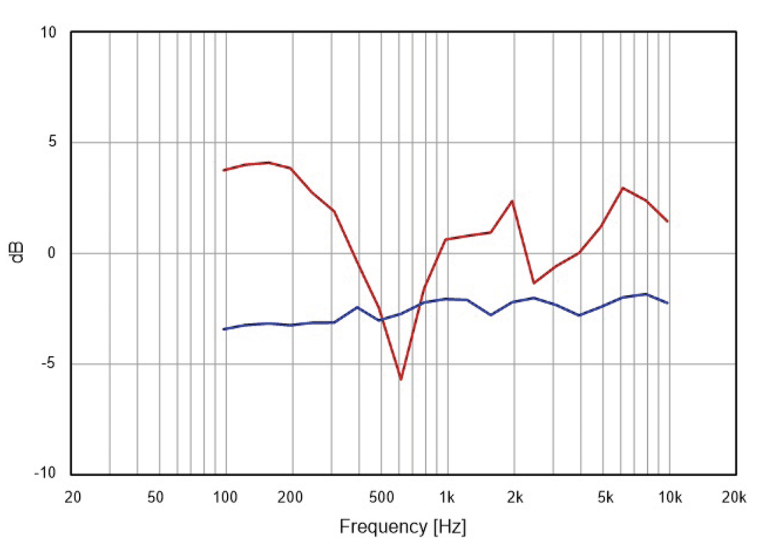
Figure 7 shows two examples of headphone L-R Tracking errors. The lower curve shows a simple sensitivity offset. The upper curve clearly reveals a problem in one channel.
Two-Tone Distortion Measurements
Harmonic distortion measurements are necessarily limited in band-pass systems (e.g., headphones). Leakage or an open low impedance design can cause overestimation of the relative harmonic distortion at low frequencies. As the fundamental frequency is increased, most harmonics will fall outside the passband. Furthermore, the upper frequency limit of the measurement system, sampling/A-to-D converter, or microphone/coupler/ear simulator will impose a limit on the highest measureable harmonic component.
Two-tone distortion measurements are generally used to overcome these limitations. The more complex stimulus signal excites higher order nonlinearities and is a better approximation of speech, music, or other signals the device may encounter in real use. Furthermore, the signal purity requirement for the generators (in terms of harmonic distortion) is relaxed, as measurements are performed at frequencies other than the harmonics of either stimulus signals. Note that difference frequency and intermodulation distortion tests are alternative methods of detecting the effects of non-linearities in a system, not different causes of nonlinear distortion.
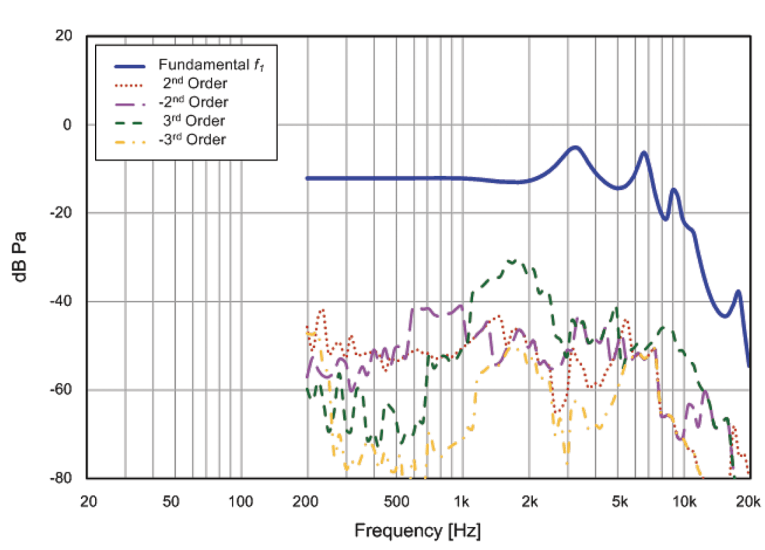
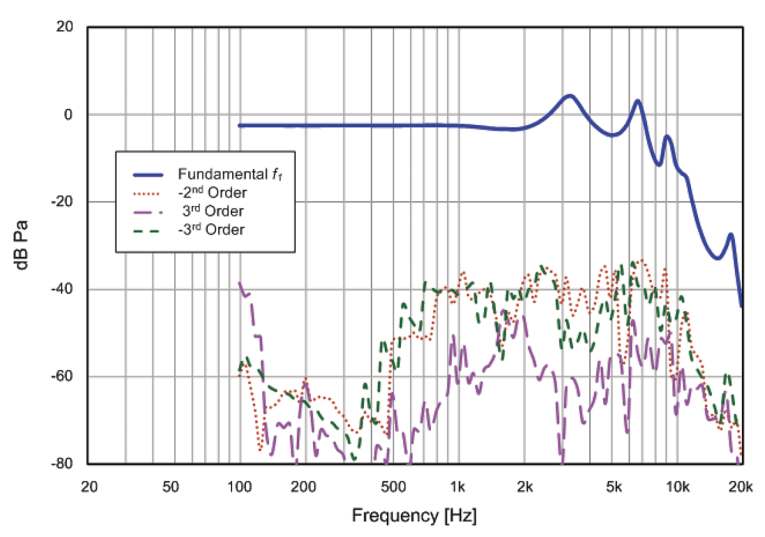
The intermodulation distortion (IMD) test uses a stepped frequency tone, f1, and a fixed low frequency tone, f2. The level of f2 is +12 dB relative to f1 (a 4:1 ratio). The frequency reference for the stimulus is f1. Sum and difference frequency components are measured and these the distortion products follow f1 as an ensemble. The interval between the distortion products is f2 [1],[2]. At a minimum, second order, (f1 + f2) and (f1 – f2), and third order, (f1 + 2f2) and (f1 – 2f2), distortion products are measured. For R40 stimulus frequencies, an IMD test using the IEC standard fixed low frequency tone of f2 = 70 Hz will start with f1 at 200 Hz in order to avoid coincidence frequencies with harmonics (see Figure 8).
Difference frequency distortion (DFD) is measured using an input signal composed of two sinusoidal signals of equal level at different frequencies, f1 > f2. The difference in frequency between the two stimulus tones, Δf = f1 – f2, is held constant as the two tones are stepped in frequency. The interval between the sum and difference frequency components is Δf. The even order distortion products remain at fixed low frequencies while the odd order distortion products follow f1 and f2 as an ensemble[1],[2]. At a minimum, the second order, (f1 – f2), and third order, (2f1 – f2) and (2f2 – f1), distortion products are measured (see Figure 9).
Conclusion
It is important to specify the input level for headphone frequency response measurements. The sensitivity can be specified at a single frequency or be plotted as a calibrated transfer function at all frequencies. Averaging of five response trials can be used to mitigate the effects of positioning and leakage for circum-aural, supra-aural, or intra-concha devices. Standardized free and diffuse field corrected responses can easily be calculated. This also ensures uniform comparative data across manufacturers or test laboratories, even if the design target is something other than diffuse or free field.
The matching between left and right channels should be calculated in a manner relevant to loudness perception, giving an accurate and easy to interpret result that correlates to actual performance. In addition, performing two-tone intermodulation and difference frequency measurements across frequencies provides a more reliable indication of nonlinear distortion in a band limited system such as a headphone. VC
This article was originally published in Voice Coil, July 2019.
References
[1] International Electrotechnical Commission (IEC) 60268-7, “Sound System Equipment—Part 7: Headphones and Headsets.”
[2] American National Standards Institute (ANSI)/Acoustical Society of America (ASA) S3.7-2016, “Method for Measurement and Calibration of Earphones.”
[3] C. J. Struck, “Refinements in the Electroacoustic Testing of Headphones,” proceedings of the Audio Engineering Society International Conference on Headphone Technology, Aalborg, Denmark, August 2016.
[4] C. J. Struck, “Evaluation of Headphone Performance with a Head And Torso Simulator,” presented at the 13th International Congress on Acoustics, Zagreb, August 1989.
[5] Institute of Electrical and Electronics Engineers (IEEE) 1652-2016, “Standard for Translating Head and Torso Simulator Measurements from the Eardrum to Other Acoustic Reference Points.”
About the Author
Christopher J. Struck is the CEO and Chief Scientist for CJS Labs, a consulting firm specializing in electroacoustics for product design and testing. He is also the Standards Director of the Acoustical Society of America (ASA). Over the past 30 years, he has held positions at Brüel & Kjær – Denmark, Charles M. Salter Associates, GN ReSound, and Tymphany Corp. He is a Fellow of the Acoustical Society of America, a Senior Member of the Institute of Electrical and Electronics Engineers (IEEE), and a Fellow and former Governor of the Audio Engineering Society (AES). He holds a degree in Electrical Engineering from the University of Wisconsin –Madison, where his studies also included electronic music composition. Chris is the author of many technical papers, application notes and articles. He has traveled extensively throughout the world, providing training and lecturing on the topics of acoustics, transducers, signal processing, and measurement techniques.







